Exchange Potential: LDA and GGA versus Exact Potential
Exchange Potential: LDA and GGA versus Exact Potential
The exact multiplicative exchange potential of density functional theory
can be obtained numerically via the optimized potential
method (OPM) [1].
The OPM results can be used to analyze the properties of approximate
density functionals for the exchange energy, as the
local density approximation (LDA) and the
generalized gradient approximation (GGA).
Below these approximations are compared with the exact potentials for
a number of systems, ranging from atoms to solids.
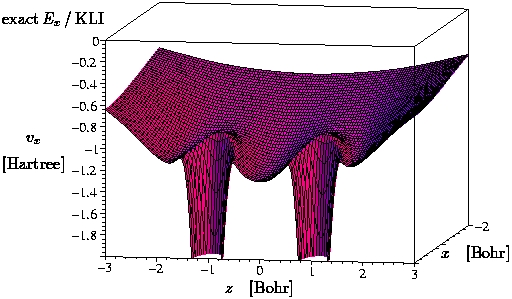
Sometimes also the Krieger-Li-Iafrate (KLI) approximation [2] to the
exact exchange potential is displayed.
The units of the plots are as follows:
All length scales are given in Bohr, the potentials are in Hartree.
II.1) Neon (GGA = PW91 [3])
-
The atomic shell structure is obvious in the exact exchange potential
(OPM).
-
The exact exchange potential falls off like -1/r for large
r, i.e. in the asymptotic region in which the density
decays exponentially.
-
The KLI approximation also shows the shell structure, although
much less pronounced.
It is exact in the asymptotic regime.
-
Neither the LDA nor the GGA reproduce the shell structure.
The LDA and GGA potentials decay exponentially for large r
(with the exception of the B88-GGA potential [4] which formally
decays as r-2 [5]).
-
The GGA potential depends on the Laplacian of the density, which
diverges like 1/r at the position of the nucleus
(cusp condition).
II.2) Chromium, Palladium, Radon
see
E. Engel and S. H. Vosko,
Phys. Rev. B 47, 13164 (1993).
II.3) Zinc
see
E. Engel and R. M. Dreizler,
J. Comput. Chem. 20, 31 (1999).
II.4) Spin-difference potentials for
Manganese, Arsenic, Copper, Europium
see
E. Engel and S. H. Vosko,
Phys. Rev. A 47, 2800 (1993).
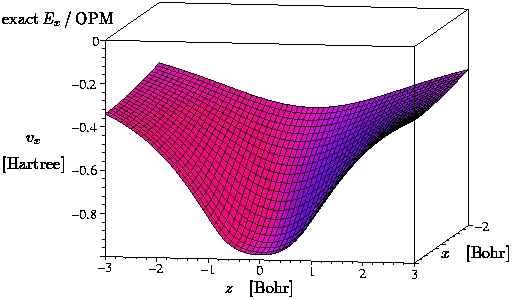
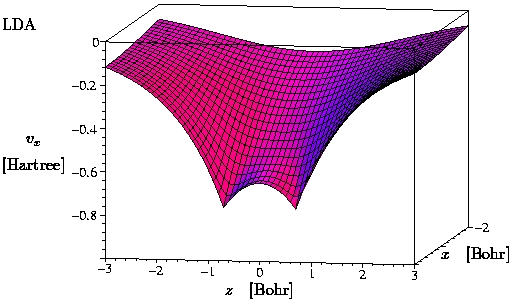
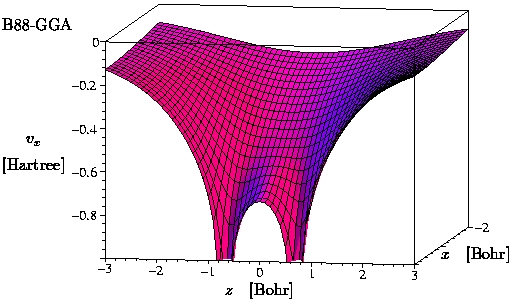
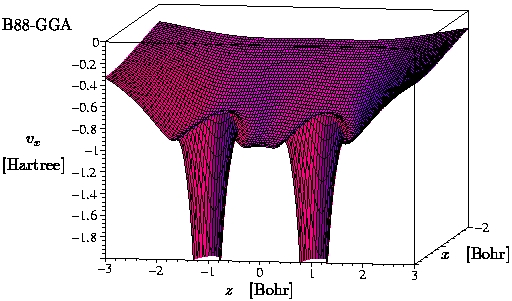
III.1) H2 (GGA = B88 [4])
-
The plots show the potential in the x-z-plane.
The atoms are sitting on the x-axis at z = +/-0.7
Bohr.
-
The exact exchange potential of H2 only
cancels the self-interaction component in the Hartree potential.
III.2) N2 (GGA = B88 [4])
-
The plots show the potential in the x-z-plane.
The atoms are sitting on the x-axis at z = +/-1.035
Bohr.
-
The shell structure is obvious in the exact potential (the KLI
approximation is rather close to the exact OPM solution as can be
verified for atoms and solids).
see
E. Engel and S. H. Vosko,
Phys. Rev. B 50, 10498 (1994).
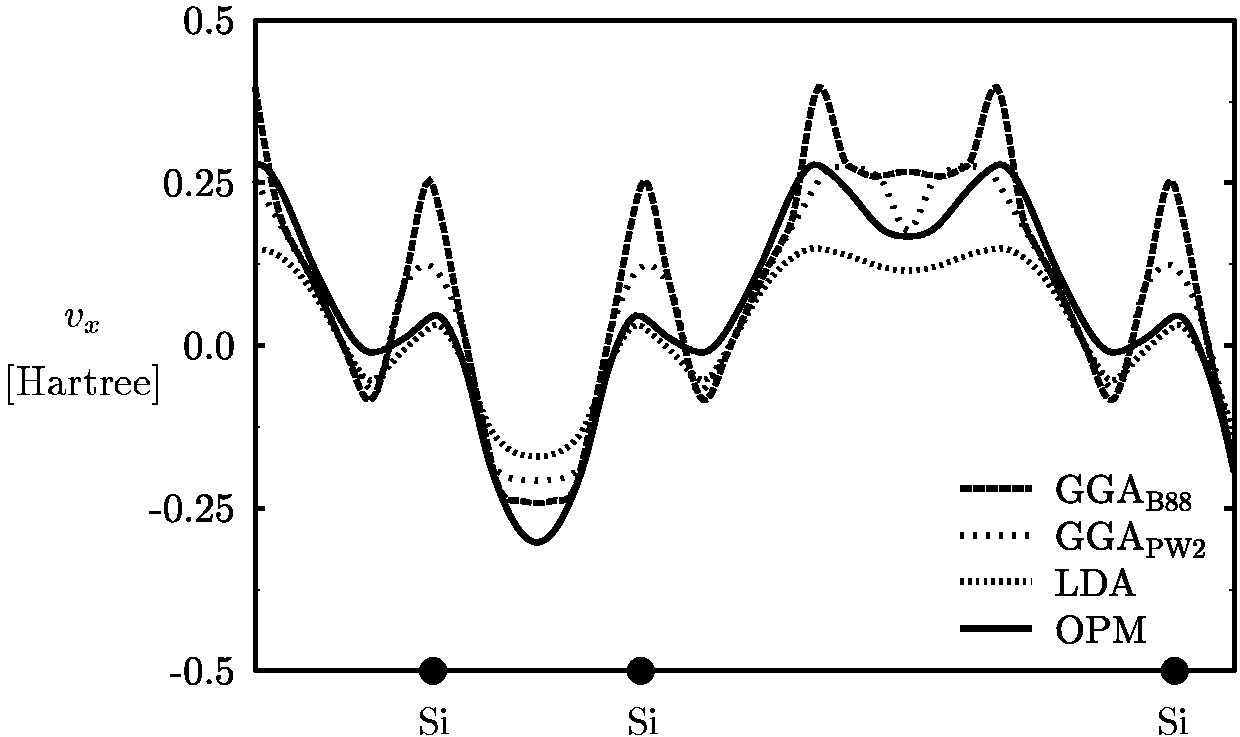
V.1) Silicon
-
GGA = PW91 [3] and B88 [4]
-
Plane-wave pseudopotential calculation with a cut-off energy of
25 Rydberg, k-point sampling based on 19 special
k-points (a = 10.2 Bohr).
-
The plots show the potential along [111] direction of the diamond
structure.
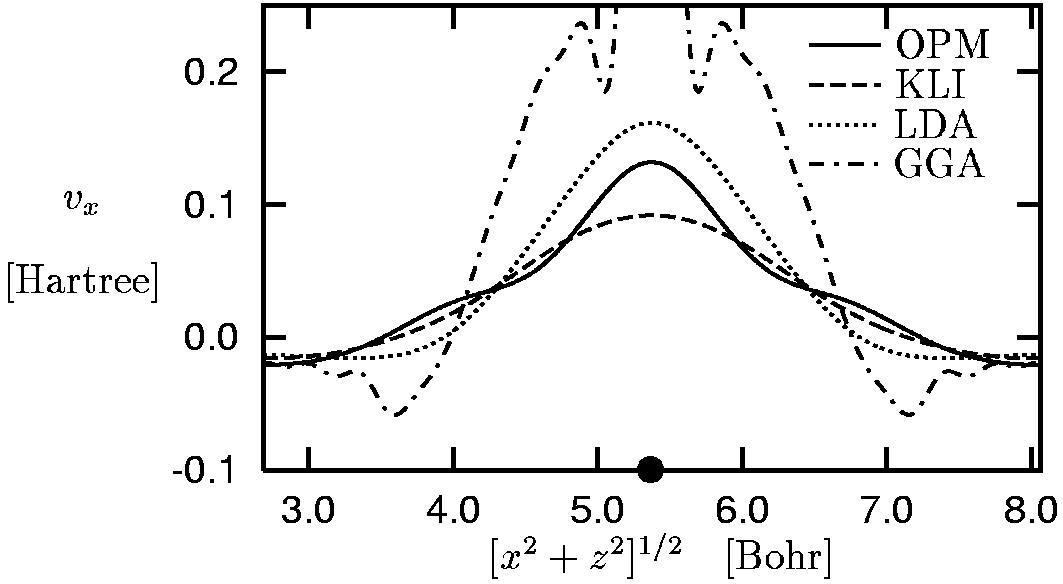
V.2) Aluminum
-
GGA = PW91 [3]
-
Plane-wave pseudopotential calculation with a cut-off energy of
100 Rydberg, k-point sampling based on 44 special
k-points, 750 Kohn-Sham states included in evaluation
of Kohn-Sham response function (a = 7.6 Bohr).
-
The plots show the potential along [100] direction of the fcc structure.
-
KLI approximation reasonably close to exact OPM result.
-
GGA deviates much more from exact potential than LDA.
-
J. D. Talman and W. F. Shadwick,
Phys. Rev. A 14, 36 (1976).
-
J. B. Krieger, Y. Li, and G. J. Iafrate,
Phys. Lett. A 146, 256 (1990).
-
J. P. Perdew, in
Electronic Structure of Solids 1991,
ed. by P. Ziesche and H. Eschrig (Akademie Verlag, Berlin, 1991),
p.11.
-
A. D. Becke,
Phys. Rev. A 38, 3098 (1988).
-
E. Engel, J. A. Chevary, L. D. Macdonald, and S. H. Vosko,
Z. Phys. D 23, 7 (1992).
Home
Top
Last modified: April 13, 2004
Disclaimer
ee